| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
THE Arabic numeral set and the decimal numerical system is the accepted standard on the planet today by which one can affirm quantity or classify a given identity. It is called the decimal system because it consists of ten elements (0 to 9) arranged in increasing powers of 10n, n ϵ I from left to right. Thus 3213.56 is no more than 3 × 103 + 2 × 102 + 1 × 101 + 3 × 100 + 5 × 10-1 + 3 × 10-2. However, the decimal system was not the first of the numerical systems employed nor by any means the more practical or best-suited. There is some belief that the decimal systems arises from the fact that human beings have ten fingers. History presents evidence that suggests the decimal system was introduced as a very deliberate and enforced standard that did not arise naturally and gain preponderance throughout the world through the process of natural selection as some historians have argued.
The first numerical system is believed to be a binary one, composed therefore, of two elements. One element we shall call A for simplicity, which represents the unit, and the second element B representing the quantity 2. (These may have been scratches of different shapes on cave walls.) Thus, in this binary system the quantity of 7 would be represented by BBBA or three B’s and one A—an additive system, unlike the modern binary system which consists of powers of base 2 and would represent 7 as 1001 (1 × 23 + 0 × 22 + 0 × 21 + 1 × 20). All quantities would be represented in a similar manner under such a system. This would do if one were keeping tracking of a small herd or counting tribe members. To represent any large quantity however would be impossible. Referring to our example, to write 3213 would require one thousand six hundred and six Bs written sequentially, followed by an A: The entire cave wall would be covered and it would take all season to scratch into the rock. As man evolved, his capacity and need for number and calculation increased. New systems were developed primarily using the fingers and toes as counting instruments. From the finger-and-toe counting came the quinine (5-base) and base-20 systems, remnants of which we still see today in many languages, as in the eighty of the French which is denoted as “four twenties” (quatre-vingts).
Among other notable systems was the 60-based system mostly used by the Mesopotamians around 1700 BC. The virtue of such systems such as the base-60 are that they are divisible by many more numbers. 60 is divisible by 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, and 30, whereas 10 is divisible only by 2 and 5. Therefore it is impossible to pay one third of a dollar and frequently for one third of any quantity. This becomes problematic if one is bartering or selling by pieces or weight. Hence the persistence of pricing by the dozen by bakers and other craftsmen and why Americans hold on to the imperial system4. Similarly, a quarter of an hour would have no meaning if clocks were on a decimal system. If you are trying to calculate fractional components of the sky for instance (astronomy) or on the clock or calendar, you will get reducible fractions and simple expressions. This means that in a base-60 system arithmetic problems worked out in even numbers much more than they would in a decimal system.
Meanwhile, in other parts of the world developments were being made parallel to those mentioned above. But it was the Hindus of India infamous for their contribution of the 0 or null element into the set of integers as well as their negative counterparts, who were elaborating upon the world’s first decimal system. This system had not been accepted readily due to the inconveniences mentioned, but somehow the Greeks came into contact with it. Pythagoras5 in the 6th century B.C. knew of it and it was partially due to his influence that the Greeks took on the decimal system as its state standard. It was not long after, through their contact with merchants and suppliers who were forced to adopt their system of counting, as well as the influence of their reputed culture, that the decimal system began to spread.
The Tetractys
Following the example of the Pythagoreans, we begin by considering the universe to be “nothingness”, devoid of all but space, a great egg, a 0. We take a point in this universe, and this is our number 1, the unity. (A point is defined as having no magnitude, only position.)
From this universe we then extract a second point. By virtue of the relationship between their respective positions of the two points, we now have a line, and what we denote by the idea “2”.6
We then take a third point outside this line and by virtue of the relationship in position between our third point and the infinite amount of points on the line (2), a plane is formed. (A plane can be defined as an infinite number of lines with proportional linear coefficients, and as a line is no more than an infinite number of points, it follows that a plane is an infinite number of points spread along a flat surface—the two-dimensional). This is the Pythagorean 3.
Taking a fourth point above this plane, and again taking this relation between the respective positions of this fourth point and all the other points in the flat surface beneath it—and a solid is formed, that is, 3-dimensional space is spanned. And it is in three dimensions that we perceive our material reality. Thus the Pythagorean 4, and it is the symbol of the physical world to the Pythagoreans for this very reason.
In summary, we have:
- One point: the field, generator of dimensions.
- Two points: generator of a line of dimension one
- Three points: generator of a triangle of dimension two
- Four points: generator of a tetrahedron, of dimension three.
The belief of the Pythagoreans was that through understanding of numbers and geometry, one could come to know Divinity.
Taking the number of 4 as representative of physical existence, they pondered on how man should reach the Unity, the source, the Indivisible Creator, the Perfect. Drawing four points on a line, and then 3 above it, and 2 above it, filtering finally to one, forming a triangle they called the tetractys:
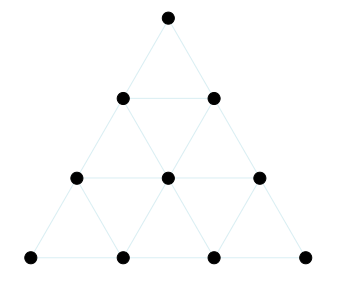
Figure 3 - The Tetractys
Notice that no matter which direction we approach the tetractys from, the four dots (the elemental or physical) are encountered first and filtered successively to the Unity. By casting off one dot at a time, the coarser becomes the finer until the source is reached. The solid collapses into the plane, the plane onto the line, and the line onto its point of origin.
The Pythagoreans took this as the symbol of what they saw as the human aspiration: the ascension from the physical to the heavenly unity. Counting the total number of points, we have 4 + 3 + 2 + 1 = 10, and so the Pythagoreans took the decimal system over all others as the system most in harmony with their purpose and humanity’s goals. One would think, that having ten fingers, for this reason alone man would have favoured the decimal system. But the decimal does not divide neatly into thirds or fourths, making commercial exchange difficult and simple division cumbersome; and the historical evidence suggests that out of this mystical consideration alone, was the decimal system adopted by the ruling classes and scholars. It subsequently found its way into Greek culture in general and in particular as a numerical standard in Athens. It was here, at the time and place of the birth of Western Civilization that through its influence as a commercial and intellectual centre and the point of gathering for merchants and scholars the world over, that the decimal system came to spread to the rest of the Western World.
4 The metric system is a decimal system: 10 millimeters makes a centimeter, 100 centimeters makes a meter, 1000 meters makes a kilometer. The imperial system is a hodge-podge of 12-base system and a 60 base system: 12 inches make a foot, 36 feet make a yard, 1760 yards make a mile. A quarter of an inch or a foot or a mile are whole integers, while a quarter of anything in the decimal system is necessarily a fractional quantity. Hence your baker would have to break bread to close the deal.
5 Anecdotally, Pythagoras was known as the “father of numbers” in his day, and is quoted as stating that “number is the ruler of form and idea and the cause of gods and demons.” He lectured his students from behind a veil and forbade everyone he knew from eating beans. They used the symbol of the pentagram (five-pointed star) to denote their order, to recognize themselves, and gain public recognition. The Pythagoreans were all vegetarians, and did not believe in personal possessions. They would help a man raise his burden onto his back but would not help him lay it down. Pythagoreans were okay to handle black cocks, but never a white cock. Despite all this, Pythagorean mathematics had a definite mystical component.
6 Mathematicians never shy of transcending logic through contradiction to remind us that a line is no more than an infinite number of points who themselves have no magnitude but when combined do. However, this is a subtlety that Pythagoreans did not pick up on at the time.